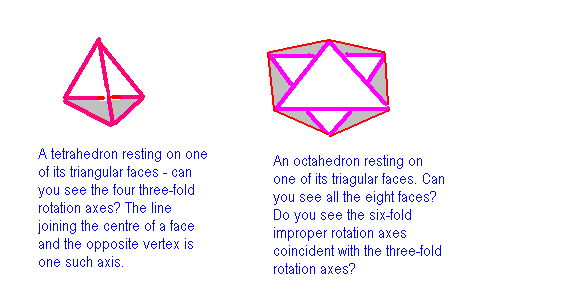
Distortions of the tetrahedron and octahedron

What happens when there is a distortion along one of the three-fold rotation axis? This is equivalent to distorting (pulling or crushing) the cube on one of its body diagonals. When this is done, two of the three C3 axes are destroyed. Such a tetrahedron will have the same symmetry as the CHCl3 molecule. The trigonally-distorted octahedron will be a trigonal anti-prism. Work out the resulting point groups.
What happens if the cube inscribing a tetrahedron is compressed down one of the cartesian axes, say the z-axis? Can you see that it destroys all the C3 axes? The cube then becomes a rectangular box. The symmetry of such a tetrahedron is same as that of the allene molecule. What is the point group? What does this distortion do to the octahedron?

Consider an octahedron where two vertices, cis to each other are made different from the other four vertices. Fe(CO)4Cl2 is such a cis-octahedral molecule. Do you see that the symmetry is same as that of H2O or CH2Cl2 molecule?
